Cartesian coordinate systems are an essential tool in mathematics, physics, engineering, and many other fields. They provide a way to describe the position of points in space using numerical values, which can be used to solve equations, plot graphs, and analyze data. In this blog, we will explore what Cartesian coordinate systems are, how they work, and why they are important.
What is a Cartesian Coordinate System?
A Cartesian coordinate system is a system of coordinates that defines the position of a point in space relative to an origin using numerical values. It was invented by the French mathematician and philosopher René Descartes in the 17th century and is named after him. The system consists of a set of two or three axes, each perpendicular to the other, intersecting at a common point called the origin. The axes are labeled with numerical values, which are used to specify the position of a point relative to the origin.
How do Cartesian Coordinate Systems work?
Cartesian coordinate systems work by using a set of two or three axes to define the position of a point in space. The axes are labeled with numerical values, usually starting from zero and increasing in value in one or both directions. The position of a point is then specified by giving its distance from the origin along each axis.
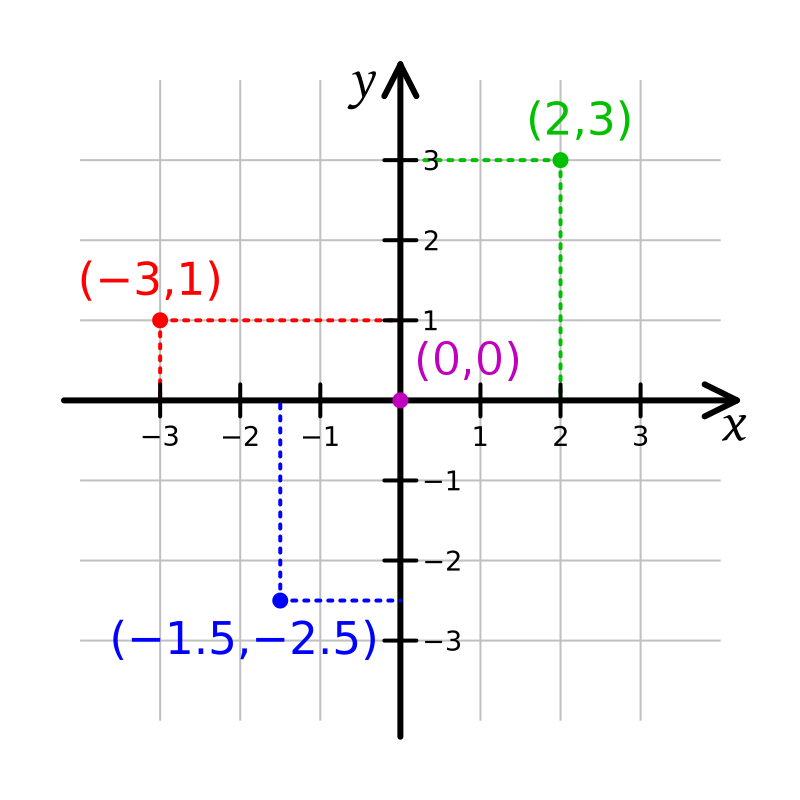
n a two-dimensional Cartesian coordinate system, there are two axes, usually labeled the x-axis and y-axis. The x-axis is usually drawn horizontally and the y-axis vertically, with both axes intersecting at the origin. The position of a point in this system is given by a pair of numerical values, (x, y), where x is the distance from the origin along the x-axis and y is the distance from the origin along the y-axis.
In a three-dimensional Cartesian coordinate system, there are three axes, usually labeled the x-axis, y-axis, and z-axis. The x-axis is usually drawn horizontally, the y-axis vertically, and the z-axis extends out of the page or screen. The position of a point in this system is given by a triplet of numerical values, (x, y, z), where x is the distance from the origin along the x-axis, y is the distance from the origin along the y-axis, and z is the distance from the origin along the z-axis.
Why are Cartesian Coordinate Systems Important?
Cartesian coordinate systems are important because they provide a way to describe the position of points in space using numerical values. This makes them useful in many areas of mathematics, physics, engineering, and other fields. For example, they can be used to:
- Solve equations: Cartesian coordinate systems can be used to solve equations that involve the position of points in space. For example, the equation of a line in a two-dimensional Cartesian coordinate system can be written as y = mx + b, where m is the slope of the line and b is the y-intercept.
- Plot graphs: Cartesian coordinate systems can be used to plot graphs of functions that involve the position of points in space. For example, the graph of a quadratic function in a two-dimensional Cartesian coordinate system is a parabola.
- Analyze data: Cartesian coordinate systems can be used to analyze data that involves the position of points in space. For example, in a three-dimensional Cartesian coordinate system, the position of a particle can be described by its x, y, and z coordinates, which can be used to study its motion and behavior.
Conclusion
Cartesian coordinate systems are a fundamental tool in mathematics, physics, engineering, and many other fields. They provide a way to describe the position of points in space using numerical values, which can be used to solve equations, plot graphs, and analyze data. By understanding how Cartesian coordinate systems work and why they are important, we can better understand the world around us and make more



